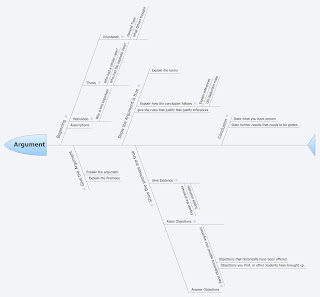
Dialectic: Intellectual Ownership
One component to the debate over Leibniz and Newton in the development of the Calculus comes with the understanding of what is meant by “owning” and idea in the academic context. Merriam- Webster defines ownership as the state, relation, or fact of being an owner. We first begin with the widely held definition of ownership and add the constraint that intellectual ownership implies the state, relation, or fact of being an owner of an idea. Obviously there exists a problem with this definition. Since we are looking at the Calculus as an idea and a development in academia the definition of ownership with regard to ‘the fact of being’ is obtuse. ‘The fact of being’ implies that there is an official declaration of owning in the form of, for example, a contract. Furthermore, ‘the fact of being’ covers material ownership, since the Calculus is not a tangible item we must reform our definition to act outside of material ownership, therefore, our working definition of intellectual ownership as the state or relation to owning and idea. By broadening our definition to ‘the state or relation’ allows us to look in what way and to what extent one owns an idea. However, now we must focus on the meaning of ‘owning’.
Owning, again defined by Merriam Webster, in the transitive form means ‘to have power or mastery over.’ That is to own, means to have authority, in our case the authority over the Calculus. Consider now the definition of intellectual ownership.
“The state or relation to have power or mastery over an idea”
Or, in layman’s terms, to have some form of authority over an idea, the clear problem now is who has “power or mastery” over an idea. For example, I have mastery over the Calculus and I am related to the Calculus is that I have studied it however; I do not have any intellectual ownership of the Calculus. To lead to a well defined definition of intellectual ownership we must now make constraints to how an individual can be in a ‘state or relation to have power or mastery over an idea.’
First the nation of ‘relation’ should be addressed. Relation here is meant by being connected by affinity, to make this definition better I shall say that one is related to an idea if the affinity between the individual and the idea if the individual developed the idea. In terms of how we define intellectual ownership this new relation implies that in order for an individual to have intellectual ownership he must have developed the idea. Even with refining our definition further we still have a problem, in that I can still be viewed as being an intellectual owner of the Calculus in that I have developed the Calculus (by means of learning it). The crux of the definition of intellectual ownership is dependent on what is implied by ‘the state’ of having ‘power or mastery.’
‘The state’ has a large context in the metaphysical properties of intellectual ownership. Since we have taken out earlier that ownership could be material or determined by an official declaration, we must consider how one can be in a ‘state’ of ‘power or mastery’ over a non-material item, such as an idea. For our worry of ‘state’ to be clarified I look toward the rules that govern academic bodies. The question that is to be answered is ‘How is one in a state of mastery or power over an idea?’ In Mathematics one is in the state of having mastery of power over an idea if the person is A) one of the developers of the idea, B) if the person made significant developments to the idea after that of the developers. Mostly the aspect of the ‘state’ is determined by the members of the academic community and who the members view to be developers of the idea. To make matters easier I will take qualifier ‘A’ as how we should define ‘state.’
NEW DEFINITION: Intellectual Ownership: The individual(s) who developed an idea at its inception and have power and mastery over the idea at the time of inception.
Note, that I have incorporated the notion of the temporal into the definition. I do this to prevent further inconsistencies in what is meant by development and developer. At this point in time we now come to a dilemma as our definition pertains to the Calculus; either Leibniz is the intellectual owner of the Calculus or Newton (note I am taking out the case of them both being the owners because they developed the Calculus independently) . Clearly, or as is taught, both Leibniz and Newton developed the Calculus. But, as was discussed earlier, the developer is greatly determined on the members of the academic group that studies idea. In that case the groups that study the Calculus are Mathematicians and Physicists.
Since the Calculus is a member of Mathematics, mathematicians are at liberty to determine who has the Intellectual Ownership over the Calculus, this means looking at Leibniz and Newton and how they developed the Calculus. As was stated in my conceptual analysis Leibniz’ contributions largely lead to the development of Mathematics while Newton leads to the foundational work in Physics. Hence:
1) Calculus is a branch of Mathematics
2) Mathematics then determines the developers of mathematical concepts.
3) Leibniz’ Calculus leads to the development of Mathematics.
4) Mathematicians view developers as people who advance Mathematics.
5) Therefore Leibniz is the developer of Mathematics
6) Which Implies that Leibniz has Intellectual Ownership of the Calculus.
Wednesday, December 8, 2010
Tuesday, October 26, 2010
Counter Example and Dilemma
Counter Example
In my analysis I gave the notational differences between Leibniz and Newton as sufficient evidence for Leibniz as the founder of mathematics. However this is only one argument that is given to legitimize this claim that Leibniz is the soul founder, other arguments include dates of publication, type of publication, and general acceptability of the men’s theories. For example while Leibniz published papers on the Calculus, Newton published the Principia Mathematica which laid out Newton’s Calculus as well has Classical Mechanics. These other arguments do, in fact, follow a common idea, one of intellectual ownership. What does it mean for a person to own an idea? This question in the ground work for a counter example that if true would make the claim that Newton is the father of Calculus regardless of how we think about the Calculus today or what Leibniz’ contributions to the advancement of mathematics were.
Consider for a moment then that Newton and Leibniz did not formulate independent views of the Calculus. In particular that Leibniz based his Calculus off of Newton’s but did so in a way that was much more metaphysical, meaning that while Newton could only describe his new mathematics in terms of English by referring to terms like velocity, Leibniz would then be able to talk about this new mathematics in terms of mathematics. That is to say that the first derivative is dy/dx. If Leibniz’ Calculus is simply a translation of Newton’s Calculus into mathematical notions then Leibniz is not the founder of the Calculus merely the man who made it understandable in mathematics.
Dilemma
Assuming my counter example that Leibniz based his Calculus not independently of Newton’s creates a dilemma in that Leibniz may then only as a developer of the Calculus. The implication then is that he did not have the background knowledge the lead to Newton’s Calculus nor Newton understanding. All that Leibniz would be able to claim, is that Leibniz simply generalized Newton’s Calculus, which is also a very notable place to be in the history of mathematics. The notion of Leibniz’ work being dependent on the work of Newton would then imply that Leibniz’ first had an understanding of the scientific and applied notion of the Calculus to which he then build the theoretical and generalized notions. Which adds to a grander dilemma that is Leibniz educational background; if Leibniz started out with the Newtonian Calculus Leibniz would have been a natural philosopher. These dilemmas lead to contradictions about Leibniz and his academic work.
In my analysis I gave the notational differences between Leibniz and Newton as sufficient evidence for Leibniz as the founder of mathematics. However this is only one argument that is given to legitimize this claim that Leibniz is the soul founder, other arguments include dates of publication, type of publication, and general acceptability of the men’s theories. For example while Leibniz published papers on the Calculus, Newton published the Principia Mathematica which laid out Newton’s Calculus as well has Classical Mechanics. These other arguments do, in fact, follow a common idea, one of intellectual ownership. What does it mean for a person to own an idea? This question in the ground work for a counter example that if true would make the claim that Newton is the father of Calculus regardless of how we think about the Calculus today or what Leibniz’ contributions to the advancement of mathematics were.
Consider for a moment then that Newton and Leibniz did not formulate independent views of the Calculus. In particular that Leibniz based his Calculus off of Newton’s but did so in a way that was much more metaphysical, meaning that while Newton could only describe his new mathematics in terms of English by referring to terms like velocity, Leibniz would then be able to talk about this new mathematics in terms of mathematics. That is to say that the first derivative is dy/dx. If Leibniz’ Calculus is simply a translation of Newton’s Calculus into mathematical notions then Leibniz is not the founder of the Calculus merely the man who made it understandable in mathematics.
Dilemma
Assuming my counter example that Leibniz based his Calculus not independently of Newton’s creates a dilemma in that Leibniz may then only as a developer of the Calculus. The implication then is that he did not have the background knowledge the lead to Newton’s Calculus nor Newton understanding. All that Leibniz would be able to claim, is that Leibniz simply generalized Newton’s Calculus, which is also a very notable place to be in the history of mathematics. The notion of Leibniz’ work being dependent on the work of Newton would then imply that Leibniz’ first had an understanding of the scientific and applied notion of the Calculus to which he then build the theoretical and generalized notions. Which adds to a grander dilemma that is Leibniz educational background; if Leibniz started out with the Newtonian Calculus Leibniz would have been a natural philosopher. These dilemmas lead to contradictions about Leibniz and his academic work.
Tuesday, October 19, 2010
Fathering the Calculus
Mathematics is the study of abstract bodies and objects that allows us to explain the universe and its abstractions work. Essentially an exercise in first order and second order logic Mathematics is a conservative discipline which since it’s foundation in the time of Euclid and Archimedes has only gone through 2 or 3 paradigmatic shifts. One of such shifts was the development of the Calculus. The Calculus, from the Latin “to count with small stones,” was developed in the late 1600’s by two men independently, Isaac Newton and Gottfried Leibniz. However, this new field has also spawned some of the greatest debate with in mathematics, and asked the question ‘who developed the Calculus?’ The debate still rages on today and is an important question in the metaphysics of mathematics. While the calculus is responsible, in large part, for most of the modern era’s scientific and technological breakthroughs it is the mind set behind it is also responsible for the progression of mathematics as a discipline. In terms of how the Calculus has advanced mathematics is what needs to be addressed, after all the Calculus is a field in mathematics. In developing the Calculus it is clear (or soon will be) the Leibniz and Newton had very different philosophies on what the Calculus was. However, regardless of who published first or which man was more popular at the time, the man with the correct thinking of what that Calculus is must then be the proverbial father of the Calculus in that this mind set would lead to the progression of mathematics.
In a nut shell the Calculus is the study of the relationship between any function, a relationship between two sets a domain and an image (or range), and how the domain related to the image. For example if one were to plot the distance traveled of a motorcycle over some time domain the Calculus looks at how changed in the time domain leads to some change in the distance image through some function. The earliest research in this question of how the domain and the image of a function related goes back to the time of Archimedes when he proved that the area under a simple parabola and a linear line cutting the parabola was proportional to the inscribed triangle between the parabola and the linear line. In the centuries that followed mathematicians learned of relationships such as the derivative (the change of the image with respect to the domain, or the set of tangent functions) and the anti- derivative (given a function g, the anti-derivative if the function f such that the derivative of f is g) but found no relationship between the two. Now enters Newton and Leibniz in the late 1600’s who both found the relationship between the derivative and the anti-derivative. Know as the Fundamental Theorem of Calculus:
First part
This part of the theorem is sometimes referred to as the First Fundamental Theorem of Calculus. A real-valued function F is defined on a closed interval [a, b] by setting, for all x in [a, b],
where ƒ is a real-valued function continuous on [a, b]. Then, F is continuous on [a, b], differentiable on the open interval (a, b), and
for all x in (a, b).
Second part
This part is sometimes referred to as the Second Fundamental Theorem of Calculus or the Newton-Leibniz Axiom. Let ƒ be a real-valued function defined on a closed interval [a, b] that admits an anti-derivative F on [a, b]. That is, ƒ and F are functions such that for all x in [a, b],
If ƒ is integrable on [a, b] then
Because it does not assume that ƒ is continuous, the Second Part is slightly stronger than the Corollary. When an anti-derivative F exists, there are infinitely many anti-derivatives for ƒ, obtained by adding an arbitrary constant to F. Also, by the first part of the theorem, anti-derivatives of ƒ always exist when ƒ is continuous.
Both Newton and Leibniz both came to the same realization, however through different understandings of the problem. Newton views functions in terms of one domain, the time domain and the functions as taking a time to a distance or a velocity or acceleration. The Calculus to Newton was no more than a means to do physics and much of his findings were done using geometric approximations. In mathematics what Newton saw did not matter, he viewed functions and the areas under the functions to have a physical meaning, i.e. that the derivative of the distance function is the velocity and the area under the velocity function over some time is the distance. We see this physical understanding of Newton’s in his notation where there is no mathematical meaning to it. He symbolized functions with the letter ‘y’ and then symbolized the velocity of ‘y’ as ‘y’ with one dot over it and two dots for the acceleration. These notations add nothing to how we in mathematics expand our understanding of mathematics, but allows for the progress of physics. These notations and equating this line of thought to moving bodies led to classical or Newtonian Mechanics. Newton was very parochial in his describing the calculus and left very vague notations which at the end of the day is not more than applied mathematics.
Leibniz, on the other hand, made much more general realizations. Instead of looking at just the time domain and the distance or the other images he looked at any two sets, one and image and one a domain. What is interesting then in the philosophy which further distinguishes Newton and Leibniz is the idea of the infinitesimal. The infinitesimal is an infinitely small area under a curve which Leibniz saw could be added up using sums. The infinite sums that Leibniz developed led to his developing the Calculus. The idea of having extremely small quantities led to the progression of mathematics which in turn has led to the modern era of mathematics (the Hilbert Paradigm) in which every statement in mathematics must be proven. Having extremely small quantities allows mathematicians to build a system in which the calculus can be proven, this system is called real analysis and is the corner stone of modern day mathematics.
Clearly both men are important individuals in mathematics and physics respectively. But in terms of the advancement of mathematics Leibniz did more to lay the foundation ground work for how mathematics is thought of then Newton did. Leibniz began mathematics on a new path where the deductive power of mathematics would separate the discipline from science where mathematics was applied. Leibniz moved mathematics to a more independent state, with his generalization of functions and domains and images the Calculus was a much more important tool than Newton could have believed. Even the notation that Leibniz used ,’dy/dx’ (meaning the change in ‘y’ over the change in ‘x’), created applications and understanding of the Calculus ranging from Political Science to Economics and of course in all three of the natural sciences. Leibniz is rightfully the father of the Calculus, he saw the generalization of the derivative and the anti-derivative in way Newton did not, he saw functions as abstract entities which Newton did not and he did indeed view the Calculus as counting by small, very small, stones.
In a nut shell the Calculus is the study of the relationship between any function, a relationship between two sets a domain and an image (or range), and how the domain related to the image. For example if one were to plot the distance traveled of a motorcycle over some time domain the Calculus looks at how changed in the time domain leads to some change in the distance image through some function. The earliest research in this question of how the domain and the image of a function related goes back to the time of Archimedes when he proved that the area under a simple parabola and a linear line cutting the parabola was proportional to the inscribed triangle between the parabola and the linear line. In the centuries that followed mathematicians learned of relationships such as the derivative (the change of the image with respect to the domain, or the set of tangent functions) and the anti- derivative (given a function g, the anti-derivative if the function f such that the derivative of f is g) but found no relationship between the two. Now enters Newton and Leibniz in the late 1600’s who both found the relationship between the derivative and the anti-derivative. Know as the Fundamental Theorem of Calculus:
First part
This part of the theorem is sometimes referred to as the First Fundamental Theorem of Calculus. A real-valued function F is defined on a closed interval [a, b] by setting, for all x in [a, b],
where ƒ is a real-valued function continuous on [a, b]. Then, F is continuous on [a, b], differentiable on the open interval (a, b), and
for all x in (a, b).
Second part
This part is sometimes referred to as the Second Fundamental Theorem of Calculus or the Newton-Leibniz Axiom. Let ƒ be a real-valued function defined on a closed interval [a, b] that admits an anti-derivative F on [a, b]. That is, ƒ and F are functions such that for all x in [a, b],
If ƒ is integrable on [a, b] then
Because it does not assume that ƒ is continuous, the Second Part is slightly stronger than the Corollary. When an anti-derivative F exists, there are infinitely many anti-derivatives for ƒ, obtained by adding an arbitrary constant to F. Also, by the first part of the theorem, anti-derivatives of ƒ always exist when ƒ is continuous.
Both Newton and Leibniz both came to the same realization, however through different understandings of the problem. Newton views functions in terms of one domain, the time domain and the functions as taking a time to a distance or a velocity or acceleration. The Calculus to Newton was no more than a means to do physics and much of his findings were done using geometric approximations. In mathematics what Newton saw did not matter, he viewed functions and the areas under the functions to have a physical meaning, i.e. that the derivative of the distance function is the velocity and the area under the velocity function over some time is the distance. We see this physical understanding of Newton’s in his notation where there is no mathematical meaning to it. He symbolized functions with the letter ‘y’ and then symbolized the velocity of ‘y’ as ‘y’ with one dot over it and two dots for the acceleration. These notations add nothing to how we in mathematics expand our understanding of mathematics, but allows for the progress of physics. These notations and equating this line of thought to moving bodies led to classical or Newtonian Mechanics. Newton was very parochial in his describing the calculus and left very vague notations which at the end of the day is not more than applied mathematics.
Leibniz, on the other hand, made much more general realizations. Instead of looking at just the time domain and the distance or the other images he looked at any two sets, one and image and one a domain. What is interesting then in the philosophy which further distinguishes Newton and Leibniz is the idea of the infinitesimal. The infinitesimal is an infinitely small area under a curve which Leibniz saw could be added up using sums. The infinite sums that Leibniz developed led to his developing the Calculus. The idea of having extremely small quantities led to the progression of mathematics which in turn has led to the modern era of mathematics (the Hilbert Paradigm) in which every statement in mathematics must be proven. Having extremely small quantities allows mathematicians to build a system in which the calculus can be proven, this system is called real analysis and is the corner stone of modern day mathematics.
Clearly both men are important individuals in mathematics and physics respectively. But in terms of the advancement of mathematics Leibniz did more to lay the foundation ground work for how mathematics is thought of then Newton did. Leibniz began mathematics on a new path where the deductive power of mathematics would separate the discipline from science where mathematics was applied. Leibniz moved mathematics to a more independent state, with his generalization of functions and domains and images the Calculus was a much more important tool than Newton could have believed. Even the notation that Leibniz used ,’dy/dx’ (meaning the change in ‘y’ over the change in ‘x’), created applications and understanding of the Calculus ranging from Political Science to Economics and of course in all three of the natural sciences. Leibniz is rightfully the father of the Calculus, he saw the generalization of the derivative and the anti-derivative in way Newton did not, he saw functions as abstract entities which Newton did not and he did indeed view the Calculus as counting by small, very small, stones.
Wednesday, September 22, 2010
DANCER
1) Take the 2 large triangle. position them such that they form a square.
2) Along the diagonal shift the triangle such that the hypotenuses over lap only half of the hypotenuses.
3) Take the medium triangle and position the hypotenuse of the medium triangle and overlap it slightly to the vertical leg of the bottom large triangle.
4) Take the 2 small triangles position the hypotenuses to the right
5) one of the move the the lowest hanging triangle such that the hypotenuse is still right. the other put next to the bottom of the lower largest triangle.
6) take the parallelogram next to the top large to make arms
7) put the square on top.
you should get a dancer.
2) Along the diagonal shift the triangle such that the hypotenuses over lap only half of the hypotenuses.
3) Take the medium triangle and position the hypotenuse of the medium triangle and overlap it slightly to the vertical leg of the bottom large triangle.
4) Take the 2 small triangles position the hypotenuses to the right
5) one of the move the the lowest hanging triangle such that the hypotenuse is still right. the other put next to the bottom of the lower largest triangle.
6) take the parallelogram next to the top large to make arms
7) put the square on top.
you should get a dancer.
More about population control...
Darwin postulated that a species reproduces at higher rates than can be maintained or sustained; humans, like all species, have this problem and reproduce at a net exponential rate. It is true that there is only a finite amount of goods that can be grown or produced each year. When there are more people than food available we run into many dilemmas. 1) Being that many poorer societies will starve since they will inevitably not have the funds to purchase food; the less food there is with respect to people eating means that the price of food will increase. 2) The increase of death due to starvation will lead to massive amounts of ailments which will over burden our hospitals to the point where medical costs will either shut down hospital or insure that the wealthiest are taken care of. 3) With food shortages the increase of crime (be it theft, murder or any other crime that will lead to the acquisition food) will lead to martial law or a higher percentage of aggressive grievances between individuals, this will limit our ability to act as a “law and order” society. Ultimately we must see the moral implications of having the population act is such a cruel manner, it is immoral to let people die and to have them revert to barbarism. Thus, it is imperative to limit the growth of people in order to secure a well fed peaceful future.
Monday, September 20, 2010
Self Deception
Self deception is intentional.
Intentional self deception contradictory because these beliefs are compartmentalized. Nor is intentional self deception self defeating because people often do things intentionally without explicit or conscious knowledge of that intention, especially after the fact. Self deception is a gradual process in which the desire to hold a false belief evolves into actually holding that belief. Intention motivates this transformation.
Intentional self deception contradictory because these beliefs are compartmentalized. Nor is intentional self deception self defeating because people often do things intentionally without explicit or conscious knowledge of that intention, especially after the fact. Self deception is a gradual process in which the desire to hold a false belief evolves into actually holding that belief. Intention motivates this transformation.
Wednesday, September 15, 2010
Revised Population Control....I DO NOT BELIEVE THIS
It is of Mathematical certainty that there will be a point where that amount that man can produce to nourish our selves will not be equal to that in which human beings need to survive. It is true that there is only a finite amount of goods that can be grown or produced each year. When there is more people than food available we run into many dilemmas. 1) Being that starvation of many poorer societies, since they will inevitably not have the funds to purchase food; the less food there is with respect to people eating means that the price of food will increase. 2) The increase of death due to starvation will lead to massive amounts of ailments which will over burden our hospitals to the point where medical costs will either shut down hospital or insure that the wealthiest are taken care of. 3) With food shortages the increase of crime (be it theft, murder or any other crime that will lead to the acquisition food) will lead to martial law or a higher percentage of aggressive grievances between individuals, this will limit our ability to act as a “law and order” society. Ultimately we must see the moral implications of having the population act is such a cruel manner, it is immoral to let people die and to have them revert to barbarism. Thus, it is imperative to limit the growth of people in order to secure a well fed peaceful future.
Sunday, September 12, 2010
Wednesday, September 8, 2010
This is also a cool way of looking at it. + why I am doing this
Socrates Shorty
In Crito, by Plato, the ancient Philosopher Socrates presents his argument for obeying the state, an argument that guided him to face his death, in 403 BCE.
Socrates Shorty
In Plato's, the ancient Philosopher Socrates presents his argument for obeying the state, an argument that guided him to face his death, in 403 BCE, after being found guilty by the state.
Tuesday, September 7, 2010
Population Control
It is of Mathematical certainty that there will be a point where that amount that man can produce to nourish will not be equal to that in which human beings need to survive. It is with truth that there is only a finite amount of goods that can be grown or produced each year. Should we have the occurrence that there exists more people than food available we run into many dilemmas. 1) Being that starvation of many poorer societies since they will inevitably not have the funds to purchase food; the less food there is with respect to people eating means that the price of food will increase. 2) The increase of death do to starvation will lead to massive amounts of ailments which will over burden our hospitals to the point where medical costs will either shut down hospital or only insure that the wealthiest are taken care of. 3) With lower food shortages the increase of crime (be it theft, murder or any other crime that will lead to the acquisition food) will lead to martial law or a higher percentage of cases between individuals, this will limit our ability to act as a “law and order” society. Ultimately we must see the moral implications of having the population act is such a cruel manner, it is immoral to let people die and to have them revert to barbarism. Thus, it is imperative to limit the growth of people in order to accommodate the food supplies of the people.
Hello I am back from Germany
Yea I went to Germany. It was fun, now I am going to use this blog for one of my Philosophy courses. Some of the entries may seem weird.
Maddrey
Maddrey
Sunday, February 7, 2010
Snowpocalypse

Friday February 5th started like any other day....until the snow came.
We went out as the snow fell, me and the international guys, and walked back out to Harvey Stone. We sled we laughed we started a fire in the fire place back there you know had a great time. I went back with everyone to the International House and in the morning this happened...
I know it looks great, the picture. At that time it had been snowing for about 24 hours, and we had, at that point, about 28 + inches. We soon got the idea that we could go to Glar and have a good meal. Which we did. But getting there was not easy.
Climbing up to Glar was a feat . The groundskeepers had not cleared a path for anything as of
 yet. So wading through what was soon becoming 30 inches of snow and as you can imagine not fun. I was at a disadvantage of wearing my six year old Adidas sneakers with holes in them. My feet were freezing and everything was on my body was wet. Even my jacket. But none the less it was fun.
yet. So wading through what was soon becoming 30 inches of snow and as you can imagine not fun. I was at a disadvantage of wearing my six year old Adidas sneakers with holes in them. My feet were freezing and everything was on my body was wet. Even my jacket. But none the less it was fun.
After breakfast we all decided to go to Lewis to see the campus and look to see is Decker Auditorium was open. This is what we saw.




After making it to Decker Lin and I went into Westminster. And that place looked pretty for a change.





Thursday, January 21, 2010
Obama's Report Card
After the first year of his Presidency it is definitely true that the populace has begun to turn against him. And I for one disagree with almost all of his policies. However this is not how we should rate a President. The President of the United States should be judged on his effectiveness, his ability to make his policies popular. In that regard I give Obama a C. I think that he is an average President, he has not, while in office, rallied the public behind health care, he was able to get the stimulus package through, which many Americans liked when he supported it and many are now running from it. We have had some economic recovery, but as a recent AP story reported the difference between districts and areas receiving government aid and those that did not was statistically in significant. And when you look at it Obama has not done much as President thus far. But overall he has been ineffective in conveying why is policies are the way to go.
Monday, January 18, 2010
Walk the walk
The political atmosphere is changing again. With a horrible first year in the Obama administration it is easy to say that trends simply favor the party out of power. However something else is happening in the Unites States, and something that many of us have not seen before. We are seeing for the first time in my life a true revolt against the government. The energy is not with any one political party but with an idea. An idea the JEFFERSON HAD IT RIGHT. We see a huge surge going back to a limited government, a small government that worked for the people, a movement to lower taxes, a movement with the 'get off my back' mentality. Essentially the mood in the country is becoming much more libertarian. Furthermore, we are seeing a conservatism such that we have never been accustomed to not since before Teddy Roosevelt.
And now what it seems to be in the political realm, is not a fight between who spends more money and who is for or not for abortions, we are seeing real debates are real concerns about the role of the federal government. This is the kind of substantive debate that is necessary for this country. For the 20 years of my life I have seen in every presidency an expansion of the government. And most Americans are not saying, Democrat and Republican alike, 'we want the government out of our lives.' This is becoming more and more evident, when people see what is going on on capitol hill. In recent years the government spent about 300 billion dollars in tax refunds to boost a recessed economy, then TARP, then the 800 Billion dollar stimulus plan to create job, then the debate on cap and trade, followed by a HUGE health care debate where in the midst of the feverish debate the congress passed an overhaul of the student loan business being controlled completely by the federal government. All of this has been done, in front of the American people, and the American people are now seeing that the left leaning authoritarian government that we have been seeing it taking too much control of our lives.
Americans are turning away in droves from the administration's and this congress' agenda. And they are turning to the individuals that are promoting the idea of basic conservative ideologies. That is limited government, lower taxes, no earmarks, strong national defense. But what is even more impressive is that people are turning against our political leaders, and they are truly understanding what it is to be American. The American people are waking up and realizing that in the formation this great republic offers 3 rights that no government have ever promised. As Edmund Burke writes America promises the right to "choose our own government" "cashier them for misconduct" and "to frame government for our selves."
However most leaders have not took to heart the words of the American people. And those leaders are now in control of congress and sitting uncomfortably in the White House. And just to fair Bush in his later years was no better, and most Republicans fell right in line. But as we see the Republicans, as the conservative party (compared to the Democrats) are attracting the Jeffersonian vision of government. And we see this EVERYWHERE, from an 11 point turn around in a years time from +9 favorable rating of congressional Democrats to not a +2 favorable rating for congressional Republicans, to the off year and special elections. The Gubernatorial races in New Jersey and Virginia showed the more conservative candidates win, but what was more impressive is that in a year's time we see the independents in these races turn in Tsunami force waves away from liberal agendas. We saw in NY-23, a Republican turned Conservative loose only by 4 points, and he would have won had there been a proper primary and not some politburo choosing the liberal Republican; Scosofatass has no business in that race and Republicans should be ashamed for her being the party pick. And now we are going to see it again in the special election for 'the peoples seat' in Massachusetts.
Scott Brown I predict will win by 5-10 points in the special election, and it is not because Coakly ran a bad campaign, it will be because the voters are choosing basic conservative values over the liberal agenda of Washington; all of the polls are showing a Brown victory. However, I have a warning for all of the Republicans:
The call the lead is coming to your ears. The American people are turning to you because they believe that you are the ones who will take the difficult conservative approach to governance. They are calling for you to do what is right, to truly be conservative and not just appear to be. It is time for all Republicans to walk the walk. Do not stray and do not dither. Be the party that emboldens all Americans to do what is right and not the party that gets elected and then sits there spends and does nothing...
And now what it seems to be in the political realm, is not a fight between who spends more money and who is for or not for abortions, we are seeing real debates are real concerns about the role of the federal government. This is the kind of substantive debate that is necessary for this country. For the 20 years of my life I have seen in every presidency an expansion of the government. And most Americans are not saying, Democrat and Republican alike, 'we want the government out of our lives.' This is becoming more and more evident, when people see what is going on on capitol hill. In recent years the government spent about 300 billion dollars in tax refunds to boost a recessed economy, then TARP, then the 800 Billion dollar stimulus plan to create job, then the debate on cap and trade, followed by a HUGE health care debate where in the midst of the feverish debate the congress passed an overhaul of the student loan business being controlled completely by the federal government. All of this has been done, in front of the American people, and the American people are now seeing that the left leaning authoritarian government that we have been seeing it taking too much control of our lives.
Americans are turning away in droves from the administration's and this congress' agenda. And they are turning to the individuals that are promoting the idea of basic conservative ideologies. That is limited government, lower taxes, no earmarks, strong national defense. But what is even more impressive is that people are turning against our political leaders, and they are truly understanding what it is to be American. The American people are waking up and realizing that in the formation this great republic offers 3 rights that no government have ever promised. As Edmund Burke writes America promises the right to "choose our own government" "cashier them for misconduct" and "to frame government for our selves."
However most leaders have not took to heart the words of the American people. And those leaders are now in control of congress and sitting uncomfortably in the White House. And just to fair Bush in his later years was no better, and most Republicans fell right in line. But as we see the Republicans, as the conservative party (compared to the Democrats) are attracting the Jeffersonian vision of government. And we see this EVERYWHERE, from an 11 point turn around in a years time from +9 favorable rating of congressional Democrats to not a +2 favorable rating for congressional Republicans, to the off year and special elections. The Gubernatorial races in New Jersey and Virginia showed the more conservative candidates win, but what was more impressive is that in a year's time we see the independents in these races turn in Tsunami force waves away from liberal agendas. We saw in NY-23, a Republican turned Conservative loose only by 4 points, and he would have won had there been a proper primary and not some politburo choosing the liberal Republican; Scosofatass has no business in that race and Republicans should be ashamed for her being the party pick. And now we are going to see it again in the special election for 'the peoples seat' in Massachusetts.
Scott Brown I predict will win by 5-10 points in the special election, and it is not because Coakly ran a bad campaign, it will be because the voters are choosing basic conservative values over the liberal agenda of Washington; all of the polls are showing a Brown victory. However, I have a warning for all of the Republicans:
The call the lead is coming to your ears. The American people are turning to you because they believe that you are the ones who will take the difficult conservative approach to governance. They are calling for you to do what is right, to truly be conservative and not just appear to be. It is time for all Republicans to walk the walk. Do not stray and do not dither. Be the party that emboldens all Americans to do what is right and not the party that gets elected and then sits there spends and does nothing...
Sunday, January 10, 2010
Subscribe to:
Posts (Atom)